Site Analysis
The choice of a proper location is the first and the very essential step in solar system design procedure. Even the most carefully planned solar system doesn’t work satisfactory, if the location wasn’t properly chosen. It is critical that the modules are exposed to sunlight without shadowing at least from 9 am to 3 pm; therefore, the properties and values of solar insolation should be studied. The modules have to be fixed with proper tilt angle allowing the system efficient operation.
Sun Path
Understanding the motion of the Sun is essential for proper solar systems design and the choice of proper location for solar collectors or photovoltaic modules. The solar path is described with the Sun path diagram. The Sun path diagram is a very useful tool in the early photovoltaic system design phase for shadowing determining. The most important geometrical parameters, which describe Earth-Sun relations, include declination (δ), sun height (α) and solar azimuth (Φ). They are defined as follows:
[Equ 1]
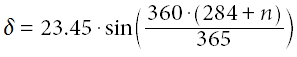
n - day of the year
[Equ 2]
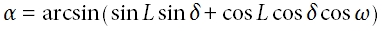
δ - declination, L - latitude, ω - hour angle[1]
[Equ 3]
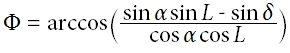
α - Sun height, L - latitude, δ - declination
Notes
| [1] | Hour angle is calculated by simple equation h = ± 15°⋅number of hours from/to solar noon. |

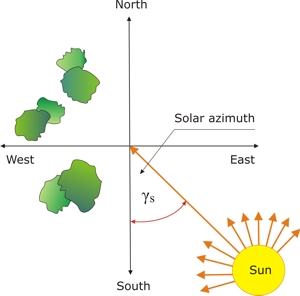
FIGURE 1: Sun height and azimuth
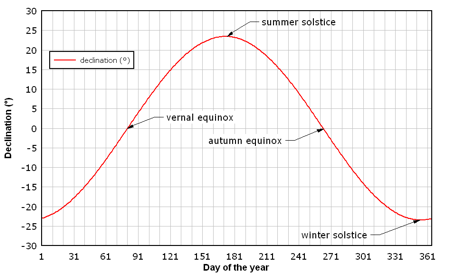
FIGURE 2: Declination, calculated by equation above, for details see literature
Sun path chart
Sun-path chart or Sun-path diagram is simple graphical method to present Sun's apparent movement. Depending on projection used following Sun path charts different sun-path charts are used:
Equidistant Sun-path chart - method was developed in 1948 by Irving F. Hand. Solar altitude lines are not geometrically projected but are equally spaced as concentric circles. This method is widely used in the USA.
Orthographic Sun-path diagram - ortographic Sun-path chart is an exact projection of sky's hemispherical vault onto 2-D plane.
Stereographic Sun-path chart - this method is easiest to draw. Drawback are dense packed lines toward diagram center so solar altitudes for high latitude locations are not easy to read from the diagram.
Gnomonic Sun-path chart - used in plain sundials also.
Cylindrical Sun-path chart - this metod is very suitable for architectural design and site/shading analysis.
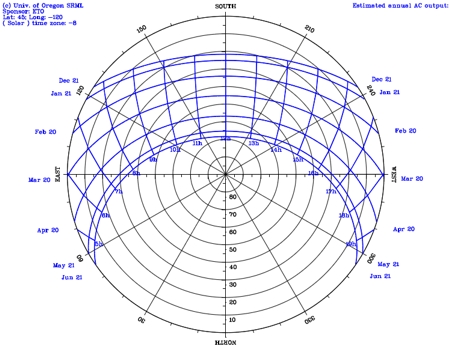
FIGURE 3: Sun-path chart, equidistant projection, generated by Sun-path Chart Software
courtesy: University of Oregon,
SRML
Tilt and Azimuth of Solar Modules
The proper tilt and azimuth angle choice is by far more important for photovoltaic systems design than solar thermal system design. Manual or automatic tilt angle adjustment can increase the total light-electricity conversion up to 30 % and more in locations with high values of solar radiation. Incidence angle should be as close to 90° as possible. Photovoltaic module tilt angle and location choice in general require more care than solar collectors tilt angle and location choice. Shaded locations, including partially shaded, are not suitable for photovoltaic module fixation. Modules should be south oriented. According to the equations above we can estimate the most proper photovoltaic modules tilt (β) employing the following equation:
[Equ 4]
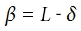
δ - declination, L - latitude
Declination changes throughout the year, therefore, the optimal tilt angle changes as well. The optimal tilt angle - at noon - for some latitudes is presented in the picture below.
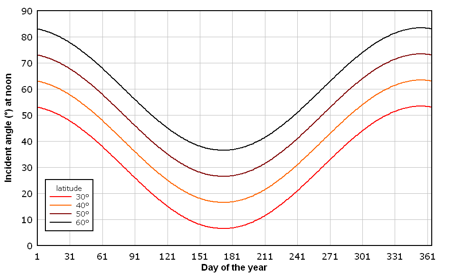
FIGURE 4: Tilt angle for latitudes 30°, 40°, 50° and 60°
The following general recommendations should be considered, if you design a photovoltaic system: Yearly average maximum output power - the photovoltaic modules tilt angle should equal local latitude. Maximum output power in winter - the photovoltaic modules tilt angle should equal local latitude + 15° (max +20°). Such a tilt angle is a good solution in areas, where the winter load is greater than the summer load. The electricity consumption for lighting is greater during winter than summer. Manual photovoltaic module tilt angle adjustment - in small systems modules should be fixed in a way, which allows manual adjustment of the module tilt angle. In March the tilt angle should be adjusted to equal latitude, in May the tilt angle equals latitude minus 10 degrees, in September the tilt angle equals latitude and in December the tilt angle equals latitude plus 10 degrees. With such an adjustment the maximal efficiency could be obtained throughout the year. Accurate and maximum energy output of larger systems should be based on exact calculations, because energy output is influenced by different factors, such as local climatic conditions (solar radiation availability in different seasons, local cloudiness or fogginess in winter, temperature and so on). You will need a long-term solar radiation data for the chosen location.
Tools for site analysis
Solar Pathfinder - The Solar Pathfinder has been the standard in the solar industry for solar site analysis for decades. Its panoramic reflection of the site instantly provides a full year of accurate solar/shade data, making it the instrument of choice. Solar Pathfinder Assistant is companion software that generates monthly sunpaths for each specific site latitude instead of relying on the stock latitude band diagrams. It includes weather data for North America, Central America, Europe, and Southwest Pacific.
SunEye™ - The Solmetric SunEye™ (discontinued) is a hand held electronic device that allows users to instantly assess total potential solar energy given the shading of a particular site. Identifying the shading pattern early in the process reduces the expense of system and home design and improves the efficiency of the final system or house.
HORIcatcher - HORIcatcher is an easy and fast tool to take outdoor pictures of the horizon. The pictures can be used to determine the solar energy input, sunshine duration and sun exposure reduced by obstacles like trees, houses or mountains. HORIcatcher is supplied with a digital camera.
Sonnenbahn Indikator Pro - is a simple tool for site evaluation.
Pilkington Sun Angle Calculator - This handy tool provides a relatively simple method of determining solar geometry variables for architectural design, such as designing shading devices or locating the position of the sun relative to a particular latitude and time.
Software for site analysis
Sun path chart - this program creates sun path charts in cartesian or polar coordinates for "typical" dates of each month (i.e.; days receiving about the mean amount of solar radiation for a day in the given month).
METEONORM - Global Meteorological Database for Solar Energy and Applied Meteorology. METEONORM is a comprehensive climatological database for solar energy applications: a meteorological database containing comprehensive climatological data for solar engineering applications at all points of the globe between the polar circles; a computer program for climatological calculations; a data source for engineering design programs in the passive, active and photovoltaic application of solar energy with comprehensive data interfaces; a standardization tool permitting developers and users of engineering design programs access to a comprehensive, uniform data basis.
Shadow Analyser - Shadow Analyzer is an advanced parametric CAD tool for professionals in the area of Solar Energy Engineering and Architecture.
Shadows - Shadows is a program used to design sundials and astrolabes and it is very useful also in solar energy engineering. Supports plane sundials with polar style of any orientation and reclination, analemmatic sundials, cylindrical and bifilar sundials. Simulates, displays and animates the shadow of the style and offers complete ephemeris of the Sun and draws the Solar Diagram.
Amethyst ShadowFX - Amethyst ShadowFX is a sun and shadow modeling program for architects and town planners. Amethyst ShadowFX enables you to easily generate shadow profiles cast by buildings and other objects for any latitude, longitude and time of year.
GOSOL - Mit dem städtebaulichen Simulationsprogramm GOSOL können städtebauliche Planungen solar+energetisch schnell und zielsicher verglichen, bewertet und optimiert werden.
Additional Information
Geomodel -
high resolution solar radiation and temperature data for any location or region, solar maps, and simulation tools for solar energy technologies (PV, CSP, CPV, solar heating/cooling).
GIS Assessment of Solar Energy Resource in Europe - On this site you can find an information on GIS-based inventory of solar energy resource and estimations of the potential photovoltaic (PV) electricity generation in Europe.
Renewable Resource Data Center - The RReDC provides information on several types of renewable energy resources in the United States, in the form of publications, data, and maps. An extensive dictionary of renewable energy related terms is also provided.
Logiciel CalSol - Estimation de l'énergie solaire disponible pour une application Energétique.
PV and snow - Peter Polder's private web site. Interesting section about snow and photovoltaic modules also included. Available in dutch language only.
Introduction to solar motion geometry - Introduction to solar motion geometry on the basis of a simple model; web site by Vyacheslav Khavrus and Ihor Shelevytsky.
PhysicalGeography.net - PhysicalGeography.net is an educational web portal that focuses on a specific area knowledge known as Physical Geography.
Sun-Earth Geometry - Books
|
|
Meeus, J. (1999), Astronomical Algorithms; Willman-Bell Inc., ISBN 0-943396-61-1. |
|
|
Lenardic, D. (2020),
Equation of Time and Analemmic Curve; Westarp, Book on Demand, ISBN 978-3-96004-072-9. Errata, First Edition (pdf). |
|
|
Muneer, T. (2004), Solar Radiation and Daylight Models, Elsevier, Butterworth-Heinemann, Oxford, ISBN 0-7506-5974-2. |
|
|
Sayigh, A.A.M., Ed. (1977), Solar Energy Engineering; Academic Press, ISBN 0-12-620850-6. |
|
|
Scharmer, K, Greif, J. (2000), The European Solar Radiation Atlas, Vol. 1: Fundamentals and maps, Presses des Mines, ISBN 2-911762-21-5. |
|
|
Scharmer, K, Greif, J. (2000), The European Solar Radiation Atlas, Vol. 2: Database and exploitation software, Presses des Mines, ISBN 2-911762-22-3. |
Sun-Earth Geometry - Papers
|
|
Reda, I., Andreas, A. (2004), Solar position algorithm for solar radiation applications; Solar Energy, vol. 76, pp 577-589. |
|
|
Reda, I., Andreas, A. (2007), Corrigendum to "Solar position algorithm for solar radiation applications"; Solar Energy, vol. 81. |
|
|
Reda, I., Andreas, A. (2008), Solar Position Algorithm for Solar Radiation Applications; NREL, NREL/TP-560-34302, January 2008. |
|
|
Blanco-Muriel et al. (2001), Computing the solar vector; Solar Energy, vol. 70, pp 431-441. |
|
|
Cooper, P. I. (1968), The absorption of radiation in solar stills; Solar Energy, vol. 12, pp 333-345. |
|
|
Grena, R. (2008), An algorithm for the computation of the solar position; Solar Energy, vol. 82, no. 5, pp 462-470. |
|
|
Walraven, R. (1978), Calculation the position of the sun; Solar Energy, vol.20, pp 393-397. |
|
|
Walraven, R. (1979), Erratum. Solar Energy vol.22, pp 195. |
|
|
Wilkinson, B. J. (1983), The effect of atmospheric refraction on the solar azimuth. Solar Energy, vol.30, pp 295. |
|
|
Archer, C. B. (1980), Comments on "Calculating the position of the sun". Solar Energy, vol.25, p 91. |
|
|
Kambezidis, H. D.; Papanikolaou, N. S. (1990), Solar position and atmospheric refraction. Solar Energy, vol. 44, pp 143-144. |
|
|
Muir, L. R. (1983), Comments on "The effect of atmospheric refraction in the solar azimuth". Solar Energy, Vol. 30, p 295. |
Other
|
|
Calculation of Sun Position, Sunrise and Sunset: Sun Position Calculator. |

